How to Find the Total Amount Paid in an Interest Rate Equation

Understanding Interest Rate Equations

Understand the terms you will be working with in your interest rate equation. When you are solving an interest rate equation, such as that for an interest rate you have for a loan you took out, you will work with several different variables. These include: P = principal amount borrowed. i = the interest rate. N = the term of the loan, in years. F = the total amount paid at the end of the designated number of years.

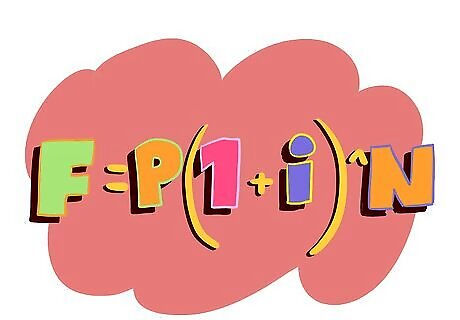
Know the equation used to calculate the total amount you will pay. To find the total amount paid at the end of the number of years you pay back your loan for, you will have to multiply the principal amount borrowed with 1 plus the interest rate. Then, raise that sum to the power of the number of years. The equation looks like this: F = P(1 + i)^N

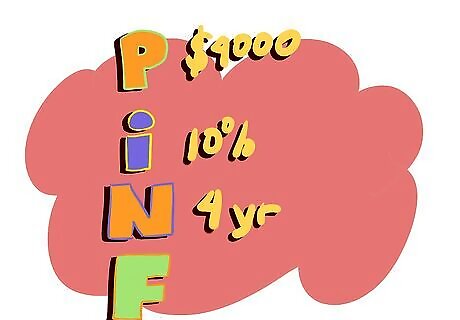
Read through the equation you are given and determine which numbers coincide with each variable of the equation. Normally, interest rate problems will be given in sentence format and you will have to figure out what each number represents. For example, you are given: “You borrow $4,000 from a bank and promise to repay the loan principal plus the accumulated interest in four years at a rate of 10% per year. How much would you repay at the end of 4 years?”. P would be $4,000. i would be 10%. N would be 4 years. F would be what you are trying to find.

Plug the known numbers into the equation for fixed rate. Once you have figured out what numbers you are working with, you can plug the numbers in so that you can work with the equation to find the fixed rate. Our equation would be: F = 4000(1 + 10%)^4. Note that to make things easier, you can convert the interest percentage to decimals so the equation would be F = 4000(1 + 0.1)^4

Solving an Interest Rate Equation to Find the Total Amount Paid

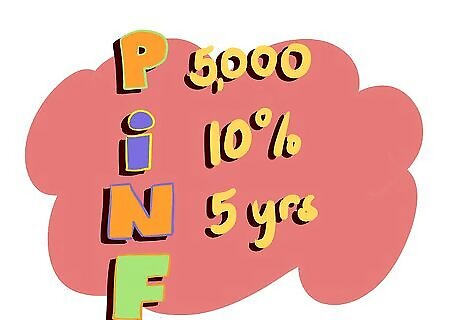
Work through the problem in stages. In order to find the total amount you will pay over the course of the time you pay back a loan, you will have to work through the article in stages. Let’s look at an example article: ”You borrow 5,000 from a bank and plan to repay the loan principal, plus and accumulated interest in five years. The rate of the interest is 10%. How much will you pay, in total, at the end of the five years?

Create your equation. Once you have read through the article, create an equation based on the standard equation F = P(1 + i)^N. For our question, our equation would be: F = 5000(1 + 0.1)^5.

Solve the inside of the parentheses first. When you have written out your equation, start to solve your problem. The first step towards doing this is to solve the equation within the parentheses first. For our equation: Solve (1 + 0.1) = 1.1. So now our equation looks like this: F = 5000(1.1)^5.

Use N to solve the next part of the equation. Once you have simplified the information in the parentheses, you should move onto applying the years (N) of the equation. This means raising the number inside the parentheses to the Nth degree. For our equation: (1.1)^5 means multiplying 1.1 to itself five times. In this case, (1.1)^5 = 1.61051.

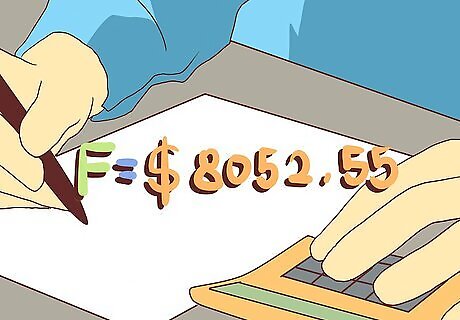
Finish the equation. You should now only have one step left in the process of solving your equation. To finish the equation and find F, or the total amount paid, you will have to multiply P with the number in the parentheses. For our equation: F = 5000(1.61051) therefore, F = $8,052.55. That means that you would have paid $8,052.55 over the course of the five years.