How to Calculate the Standard Deviation of a Portfolio

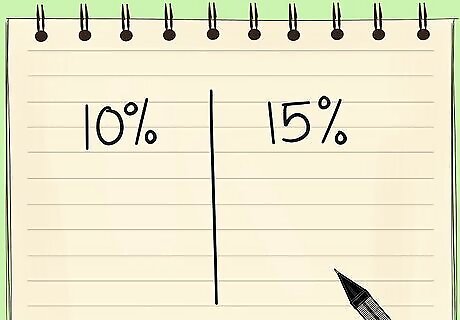
Calculate the standard deviation of each security in the portfolio. First we need to calculate the standard deviation of each security in the portfolio. You can use a calculator or the Excel function to calculate that. Let's say there are 2 securities in the portfolio whose standard deviations are 10% and 15%.

Determine the weights of securities in the portfolio. We need to know the weights of each security in the portfolio. Let's say we've invested $1000 in our portfolio of which $750 is in security 1 and $250 is in security 2. So the weight of security 1 in portfolio is 75% (750/1000) and the weight of security 2 in portfolio is 25% (250/1000).

Find the correlation between two securities. Correlation can be defined as the statistical measure of how two securities move with respect to each other. Its value lies between -1 and 1. -1 implies that the two securities move exactly opposite to each other and 1 implies that they move in exactly the same way in same direction. 0 implies that there is no relation as of how the securities move with respect to each other. For our example, let's take correlation as 0.25 which means that if one security increases by $1, the other increases by $0.25.

Calculate the variance. Variance is the square of standard deviation. For this example, variance would be calculated as (0.75^2)*(0.1^2) + (0.25^2)*(0.15^2) + 2*0.75*0.25*0.1*0.15*0.25 = 0.008438.

Calculate standard deviation. Standard deviation would be square root of variance. So, it would be equal to 0.008438^0.5 = 0.09185 = 9.185%.

Interpret the standard deviation. As we can see that standard deviation is equal to 9.185% which is less than the 10% and 15% of the securities, it is because of the correlation factor: If correlation equals 1, standard deviation would have been 11.25%. If correlation equals 0, standard deviation would have been 8.38%. If correlation equals -1, standard deviation would have been 3.75%.